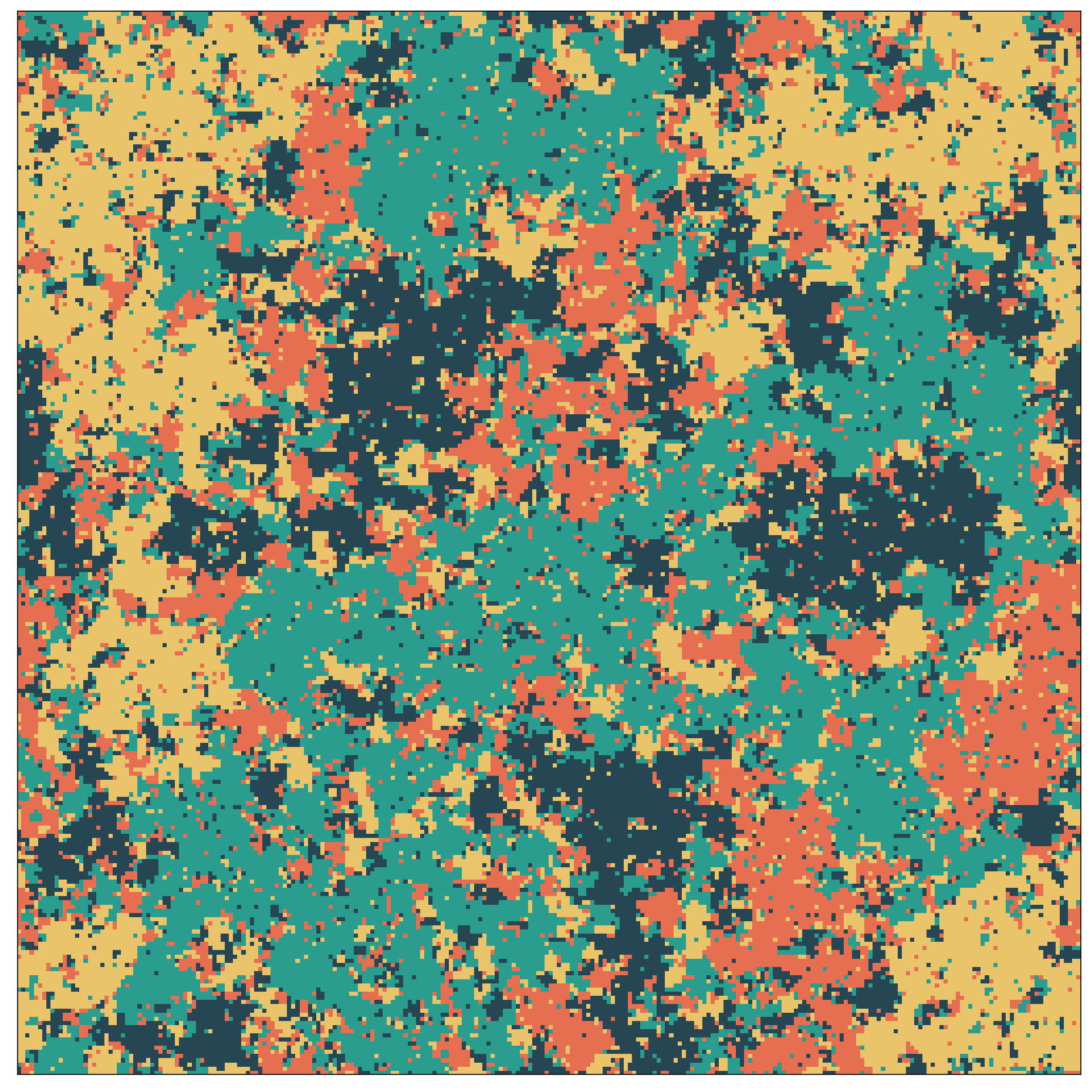
Figure 1. Critical configuration of the 4-state Potts model.
The \( q \)-state Potts model1 represents a generalization of the Ising model, where spins can take on \( q \) values, rather than only two. The Hamiltonian is given by
\[ \mathcal{H} = -J\sum\limits_{\langle i,j\rangle}\delta_{\phi_i,\phi_j}, \]
where \(J>0\), \( \phi_i\in{0,1,\ldots,q-1} \) and \( \delta \) represents the Kronecker delta. In 2D, the ferromagnetic Potts model is known to exhibit a second-order phase transition for \(q \leq 4 \) and a first-order phase transition for \( q\geq 5 \).
What makes the Potts model particularly interesting is the fact that its critical properties can be calculated exactly2. The transition temperature is, for example given by the expression
\[ \beta_c(q) = \frac{1}{2}\log\left(1+\sqrt{q}\right) \]
and the critical exponents are simple fractions, such as \( \nu = 2/3 \) and \( \beta = 1/12 \) for \( q=4 \). We verify these exponents by simulating the model using MARQOV, resulting in the following data collapse of the magnetization, which is given by
\[ \langle m \rangle = \frac{q\max{n_i}-N}{N(q-1)} \]
where \( n_i \leq N \) denotes the number of spin with configuration \(i = 1,\ldots, q \). Even though we invested only comparably little CPU time in this example, the data collapse turns out very convincing.

Figure 2. Scaling collapse of the magnetization for the 4-state Potts model.